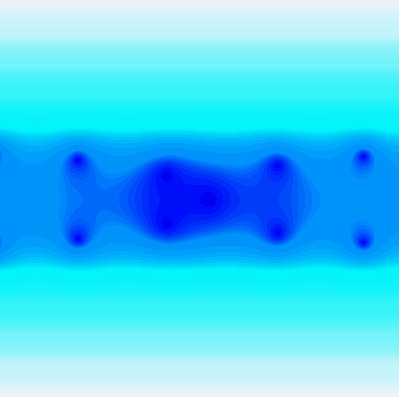
 Displacement of the Z-plane: |F(z)-z| F(z)=Cos(z) z = x + iy n=1 |x|,|y|<15 Black points are fixed points (Cos(z)=z). As color lightens, points are moved futher away from their initial positions under a single application of F(z). |
| Mathematicae Varietas |
 Displacement of the Z-plane: |F(z)-z| F(z)=Cos(z) z = x + iy n=1 |x|,|y|<15 Black points are fixed points (Cos(z)=z). As color lightens, points are moved futher away from their initial positions under a single application of F(z). |
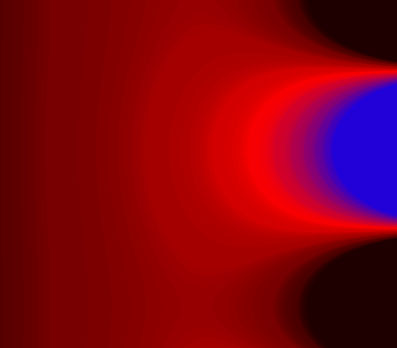 Simple Complex Dynamics Sensitive Dependence on Initial Conditions F(z)=Exp(z) z=x+iy n=2 |x|,|y|<4 Points close together remain close through two iterations (black) or move apart increasingly (red to blue) |
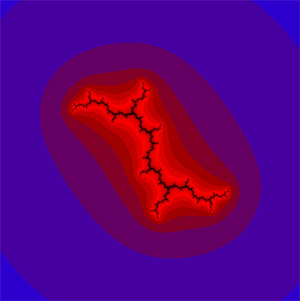 A Dendrite fractal The Julia Set arising from the complex dynamics of the function F(z)=z^2+i |
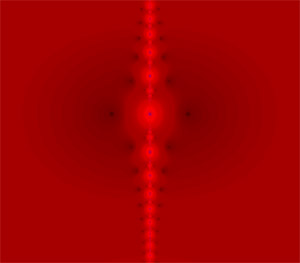 The Crimson Spine Simple Complex Dynamics Sensitive Dependence on Initial Conditions F(z)=z+1/z z=x+iy n=15 |x|,|y|<4 Darker color indicates points close together stay relatively close under iteration. Lighter shades indicate divergence. |